 |  Statistics for the Behavioral Sciences, 4/e Michael Thorne,
Mississippi State University -- Mississippi State
Martin Giesen,
Mississippi State University -- Mississippi State
Two-Way Analysis of Variance
Problems- For the problems in this chapter, assume that the within-group variability is small enough that any between-group mean differences will result in a significant F ratio. For each of the following tables, draw a graph and use it to predict the result of each tested main effect and the interaction.
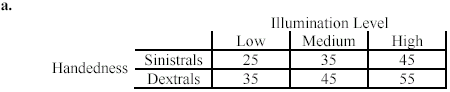
 <a onClick="window.open('/olcweb/cgi/pluginpop.cgi?it=gif:: ::/sites/dl/free/0072832517/55321/c12_img1a.gif','popWin', 'width=NaN,height=NaN,resizable,scrollbars');" href="#"><img valign="absmiddle" height="16" width="16" border="0" src="/olcweb/styles/shared/linkicons/image.gif"> (2.0K)</a> <a onClick="window.open('/olcweb/cgi/pluginpop.cgi?it=gif:: ::/sites/dl/free/0072832517/55321/c12_img1a.gif','popWin', 'width=NaN,height=NaN,resizable,scrollbars');" href="#"><img valign="absmiddle" height="16" width="16" border="0" src="/olcweb/styles/shared/linkicons/image.gif"> (2.0K)</a> 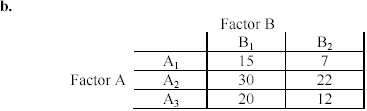 <a onClick="window.open('/olcweb/cgi/pluginpop.cgi?it=gif:: ::/sites/dl/free/0072832517/55321/c12_img1b.gif','popWin', 'width=NaN,height=NaN,resizable,scrollbars');" href="#"><img valign="absmiddle" height="16" width="16" border="0" src="/olcweb/styles/shared/linkicons/image.gif"> (3.0K)</a> <a onClick="window.open('/olcweb/cgi/pluginpop.cgi?it=gif:: ::/sites/dl/free/0072832517/55321/c12_img1b.gif','popWin', 'width=NaN,height=NaN,resizable,scrollbars');" href="#"><img valign="absmiddle" height="16" width="16" border="0" src="/olcweb/styles/shared/linkicons/image.gif"> (3.0K)</a> 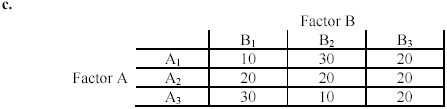 <a onClick="window.open('/olcweb/cgi/pluginpop.cgi?it=gif:: ::/sites/dl/free/0072832517/55321/c12_img1c.gif','popWin', 'width=NaN,height=NaN,resizable,scrollbars');" href="#"><img valign="absmiddle" height="16" width="16" border="0" src="/olcweb/styles/shared/linkicons/image.gif"> (3.0K)</a> <a onClick="window.open('/olcweb/cgi/pluginpop.cgi?it=gif:: ::/sites/dl/free/0072832517/55321/c12_img1c.gif','popWin', 'width=NaN,height=NaN,resizable,scrollbars');" href="#"><img valign="absmiddle" height="16" width="16" border="0" src="/olcweb/styles/shared/linkicons/image.gif"> (3.0K)</a> - A pursuit-rotor task is performed by either sinistral or dextral men (left-handed or right-handed, respectively) under three different illumination levels. For each of the hypothetical outcomes, what result would you predict for the F ratios? Cell scores are the average times on target in seconds for a 1-minute test; Ng = 10.
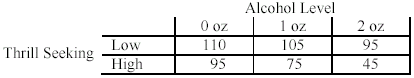
 <a onClick="window.open('/olcweb/cgi/pluginpop.cgi?it=gif:: ::/sites/dl/free/0072832517/55321/c12_img2a.gif','popWin', 'width=NaN,height=NaN,resizable,scrollbars');" href="#"><img valign="absmiddle" height="16" width="16" border="0" src="/olcweb/styles/shared/linkicons/image.gif"> (4.0K)</a> <a onClick="window.open('/olcweb/cgi/pluginpop.cgi?it=gif:: ::/sites/dl/free/0072832517/55321/c12_img2a.gif','popWin', 'width=NaN,height=NaN,resizable,scrollbars');" href="#"><img valign="absmiddle" height="16" width="16" border="0" src="/olcweb/styles/shared/linkicons/image.gif"> (4.0K)</a>  <a onClick="window.open('/olcweb/cgi/pluginpop.cgi?it=gif:: ::/sites/dl/free/0072832517/55321/c12_img2b.gif','popWin', 'width=NaN,height=NaN,resizable,scrollbars');" href="#"><img valign="absmiddle" height="16" width="16" border="0" src="/olcweb/styles/shared/linkicons/image.gif"> (4.0K)</a> <a onClick="window.open('/olcweb/cgi/pluginpop.cgi?it=gif:: ::/sites/dl/free/0072832517/55321/c12_img2b.gif','popWin', 'width=NaN,height=NaN,resizable,scrollbars');" href="#"><img valign="absmiddle" height="16" width="16" border="0" src="/olcweb/styles/shared/linkicons/image.gif"> (4.0K)</a>  <a onClick="window.open('/olcweb/cgi/pluginpop.cgi?it=gif:: ::/sites/dl/free/0072832517/55321/c12_img2c.gif','popWin', 'width=NaN,height=NaN,resizable,scrollbars');" href="#"><img valign="absmiddle" height="16" width="16" border="0" src="/olcweb/styles/shared/linkicons/image.gif"> (4.0K)</a> <a onClick="window.open('/olcweb/cgi/pluginpop.cgi?it=gif:: ::/sites/dl/free/0072832517/55321/c12_img2c.gif','popWin', 'width=NaN,height=NaN,resizable,scrollbars');" href="#"><img valign="absmiddle" height="16" width="16" border="0" src="/olcweb/styles/shared/linkicons/image.gif"> (4.0K)</a> - A study examined the effects of task difficulty and anxiety level on problem-solving ability. There were three levels of task difficulty (easy, moderate, hard) and three levels of anxiety as determined by scores on the Taylor Manifest Anxiety Scale (low, medium, high). The dependent variable was the average time to solve 10 problems in minutes. Using the following table, graph the results and indicate the probable outcome of F tests. Each cell value is a group average in minutes; Ng = 20.
 <a onClick="window.open('/olcweb/cgi/pluginpop.cgi?it=gif:: ::/sites/dl/free/0072832517/55321/c12_img3.gif','popWin', 'width=NaN,height=NaN,resizable,scrollbars');" href="#"><img valign="absmiddle" height="16" width="16" border="0" src="/olcweb/styles/shared/linkicons/image.gif"> (5.0K)</a> <a onClick="window.open('/olcweb/cgi/pluginpop.cgi?it=gif:: ::/sites/dl/free/0072832517/55321/c12_img3.gif','popWin', 'width=NaN,height=NaN,resizable,scrollbars');" href="#"><img valign="absmiddle" height="16" width="16" border="0" src="/olcweb/styles/shared/linkicons/image.gif"> (5.0K)</a> - A cognitive psychologist studied the effects of mathematics anxiety on the speed of solution of arithmetic problems. Students were tested on the Mathematics Anxiety Rating Scale and separated into high, medium, and low mathematics anxiety groups. Half of each group then solved easy (one-digit) addition problems and the other half solved hard (two-digit) problems. Each cell contains the average number of seconds taken to solve each problem. Graph the means, and predict the outcome of the significance tests.
 <a onClick="window.open('/olcweb/cgi/pluginpop.cgi?it=gif:: ::/sites/dl/free/0072832517/55321/c12_img4.gif','popWin', 'width=NaN,height=NaN,resizable,scrollbars');" href="#"><img valign="absmiddle" height="16" width="16" border="0" src="/olcweb/styles/shared/linkicons/image.gif"> (4.0K)</a> <a onClick="window.open('/olcweb/cgi/pluginpop.cgi?it=gif:: ::/sites/dl/free/0072832517/55321/c12_img4.gif','popWin', 'width=NaN,height=NaN,resizable,scrollbars');" href="#"><img valign="absmiddle" height="16" width="16" border="0" src="/olcweb/styles/shared/linkicons/image.gif"> (4.0K)</a> - A social psychologist is interested in the effects of thrill-seeking tendency and alcohol dose on performance on a simulated driving task. She administers a test that measures thrill-seeking tendency and divides the subject pool into two levels on the basis of subject scores: low and high. Thirty volunteers from each level are given either no alcohol, 1 ounce of alcohol, or 2 ounces of alcohol. After 15 minutes, each participant is tested on the task; the score is the length of time the car stays on the road in a 2-minute test. Each cell value is the average in seconds for 10 participants. Graph the results, and predict the outcome of significance tests.
 <a onClick="window.open('/olcweb/cgi/pluginpop.cgi?it=gif:: ::/sites/dl/free/0072832517/55321/c12_img5.gif','popWin', 'width=NaN,height=NaN,resizable,scrollbars');" href="#"><img valign="absmiddle" height="16" width="16" border="0" src="/olcweb/styles/shared/linkicons/image.gif"> (3.0K)</a> <a onClick="window.open('/olcweb/cgi/pluginpop.cgi?it=gif:: ::/sites/dl/free/0072832517/55321/c12_img5.gif','popWin', 'width=NaN,height=NaN,resizable,scrollbars');" href="#"><img valign="absmiddle" height="16" width="16" border="0" src="/olcweb/styles/shared/linkicons/image.gif"> (3.0K)</a> - Hypothetical F ratios for each part of Problem 2 are shown next. Give the critical value for each F ratio and provide an interpretation for each significant test. For example, a significant F ratio for handedness would mean that time on target was affected by the hand used. Review of the group means would suggest whether left-handed or right-handed participants performed better.
| Source | F | p |
| | Between groups | | | | A (handedness) | 6.31 (df = 1, 54) | | | B (illumination) | 4.05 (df = 2, 54) | | | A × B | 1.18 (df = 2, 54) | |
| Source | F | p |
| | Between groups | | | | A (handedness) | 4.52 (df = 1, 54) | | | B (illumination) | 0.67 (df = 2, 54) | | | A × B | 10.91 (df = 2, 54) | |
| Source | F | p |
| | Between groups | | | | A (handedness) | 0.75 (df = 1, 54) | | | B (illumination) | 1.18 (df = 2, 54) | | | A × B | 9.83 (df = 2, 54) | |
 Click here to view the answers (82.0K) Click here to view the answers (82.0K) |
|



 2003 McGraw-Hill Higher Education
2003 McGraw-Hill Higher Education







